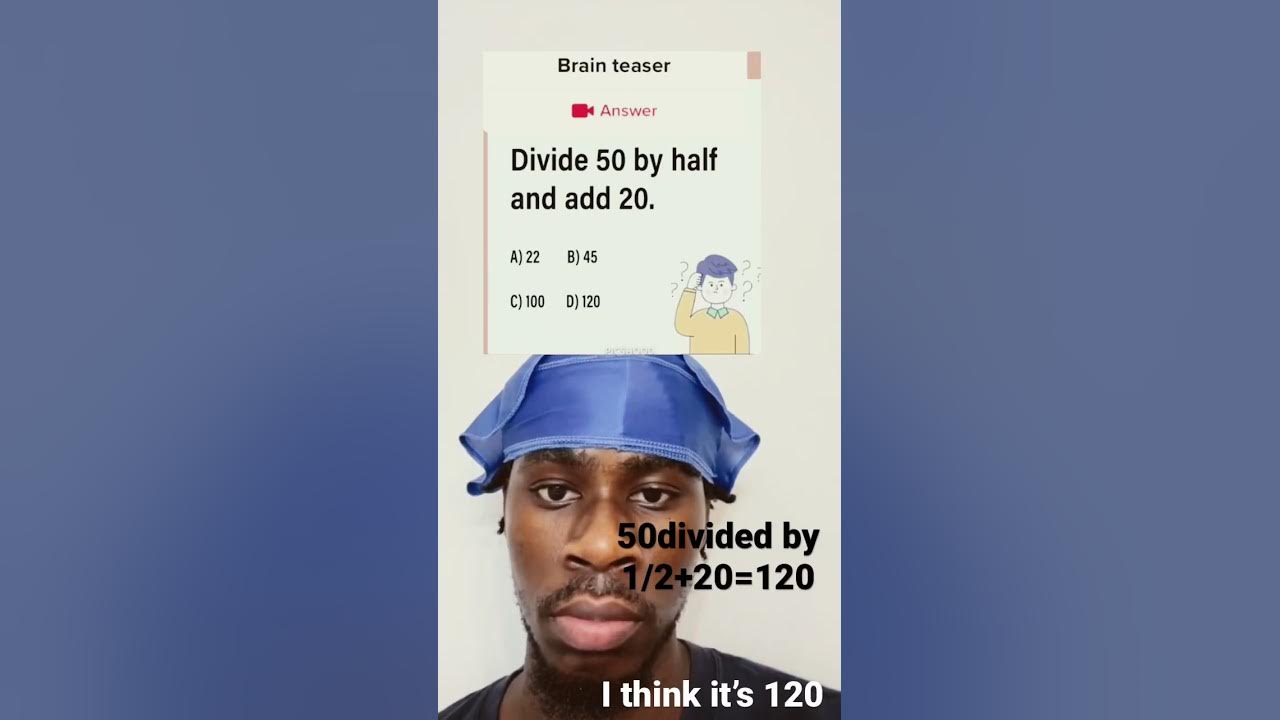
Mathematics often presents us with intriguing puzzles that not only challenge our intellect but also enhance our problem-solving skills. One such puzzle is the operation of dividing numbers and manipulating them to achieve a desired result. In this article, we will explore the expression "divide 50 by half and add 20," breaking it down step by step to unveil the solution and its implications. Understanding this operation is not just about reaching a numerical answer; it's about grasping the logic behind the arithmetic.
At first glance, the instruction to "divide 50 by half and add 20" might seem straightforward, but it requires a deeper examination of what it means to divide by half. Many people may misinterpret the operation, leading to confusion. Are we truly understanding the mathematical principles at play? What does it mean to divide a number by a fraction? These are the questions we will address, guiding you through the fascinating journey of numbers and operations.
In the following sections, we will dissect the steps involved in dividing 50 by half, adding 20 to the result, and uncover the underlying logic. We will also explore some practical applications of this operation in real-life scenarios, demonstrating that math is not just an abstract concept but a tool we can use every day. So, let's dive in and unravel the mystery behind "divide 50 by half and add 20."
What Does it Mean to Divide 50 by Half?
To understand "divide 50 by half and add 20," we first need to clarify what dividing by half entails. When we divide a number by a fraction like 1/2, we are essentially asking how many halves fit into that number. In mathematical terms, dividing by a fraction is the same as multiplying by its reciprocal. Therefore, dividing 50 by 0.5 (which is the decimal form of 1/2) means we are multiplying 50 by 2.
Step-by-Step Calculation: How to Divide 50 by Half?
The calculation can be broken down into simple steps:
- Identify the operation: Divide 50 by half (1/2).
- Convert the fraction into a decimal: 1/2 = 0.5.
- Perform the division: 50 ÷ 0.5 is the same as 50 × 2.
- Calculate the result: 50 × 2 = 100.
What Happens After Dividing 50 by Half?
Once we have divided 50 by half and obtained the result of 100, the next step is to add 20 to this outcome. This addition is straightforward and simply involves increasing the previous result by 20.
How to Add 20 to the Result of the Division?
Continuing from our previous calculation, we take the result of 100 and add 20:
- Start with the result from the division: 100.
- Add 20: 100 + 20.
- Calculate the final result: 100 + 20 = 120.
What is the Final Result of Dividing 50 by Half and Adding 20?
After following the necessary steps, we find that the final result of "divide 50 by half and add 20" is 120. This simple operation, while seemingly basic, illustrates the importance of understanding the order of operations and the meaning behind dividing by fractions.
Why is Understanding This Operation Important?
Understanding how to divide 50 by half and add 20 is not merely about reaching the correct answer. It encapsulates a broader concept in mathematics that can be applied to various real-world situations. Here are some reasons why mastering this operation is beneficial:
- Enhances problem-solving skills.
- Improves numerical literacy.
- Prepares individuals for more complex mathematical concepts.
- Encourages logical thinking and reasoning.
What Are Some Real-Life Applications of This Calculation?
Mathematics is not confined to classrooms. The operation of "divide 50 by half and add 20" can manifest in everyday situations. For instance, consider budgeting scenarios, portioning ingredients in cooking, or even calculating discounts during shopping. Understanding how to manipulate numbers fluidly allows individuals to make informed decisions and enhances their analytical capabilities.
Can This Operation Be Applied in Business Scenarios?
Absolutely! In business, calculations involving divisions and additions are routine. For example, if a company has a budget of $50,000 and decides to allocate half of it to a project while adding a bonus of $20,000 for incentives, the operation becomes crucial in determining the total budget for that project. Mastering these operations can lead to better financial planning and management.
What Other Mathematical Concepts Can We Explore?
Once you grasp the operation of dividing 50 by half and adding 20, you can delve into other mathematical principles that build upon this foundation. Concepts such as percentages, ratios, and algebraic equations all stem from similar reasoning. By strengthening your understanding of basic arithmetic, you pave the way for tackling more advanced topics with confidence.
Conclusion: Embracing the Beauty of Mathematics
In conclusion, the operation to "divide 50 by half and add 20" may seem simple, but it embodies a world of mathematical logic and reasoning. By breaking down the steps involved, we not only arrive at a numerical answer of 120 but also enhance our understanding of how numbers interact. Whether in practical scenarios, business decisions, or academic pursuits, the ability to manipulate numbers effectively is a crucial skill that can open doors to numerous opportunities.
So, the next time you encounter a mathematical operation, remember the journey of "divide 50 by half and add 20" and embrace the beauty of numbers!